Clarity on Antarctic sea ice.

I’ve always been a skeptic when it comes to Antarctic sea ice. I’m not referring here to the tiresome (and incorrect) claim that the expansion of sea ice around Antarctica somehow cancels out the dramatic losses of sea ice in the Arctic (NB: polar bears don’t really care if there is sea ice in Antarctica or not). Rather, I’m referring to the idea that the observation of Antarctic sea ice expansion represents a major conundrum in our understanding of the climate system, something one hears even from knowledgeable commentators. In this post, I’ll try to provide some clarity on this subject, with some basic background and discussion of a couple of important recent papers.

In general, Antarctic sea ice forms near the coastline, where upwelling waters cool to the atmosphere. It melts when the winds and currents push it into areas of warmer water to the north. In the summer, it melts pretty much all the way back to the coast. An efficient way to form lots of Antarctic sea ice during the autumn growth season is to have strong winds that push the ice away from the coastline. Pushing sea ice away leaves open water that can lose heat to the atmosphere, creating more sea ice. The persistent circumpolar westerlies are critical in pushing ice toward the north, into warmer waters. (Owing to the Coriolis effect, westerly winds cause northward-flowing surface ocean currents in the Southern Hemisphere).

The importance of the winds in controlling Antarctic sea ice leads to the obvious idea that changing winds can explain the increase that has been observed over the last several decades. There has indeed been a substantial increase in the circumpolar westerlies; this is very well established from observations and is associated with the oft-discussed increase in the “Southern Annular Mode” (SAM) index². Averaged over the year, the SAM index has increased nearly monotonically since the 1970s (e.g., Marshall et al., 2003). This has led to a fairly simple logic in explaining the recent sea ice increase: the westerly winds have increased, so sea ice has increased too. Furthermore, there is good evidence that the increasing westerlies are a response to anthropogenic climate forcing from CO₂ and other greenhouse gas increases in the troposphere, along with ozone declines in the stratosphere (Thompson and Solomon, 2002; Thompson et al., 2011). This would suggest that the observed increase in Antarctic sea ice extent is anthropogenic in origin, just like the Arctic sea ice decline, but for very different reasons. In short, reduced ozone in the stratosphere, and increased CO₂ in the troposphere — both climate forcings that are unequivocally anthropogenic — cause increased westerly winds, which cause Antarctic sea ice to expand.

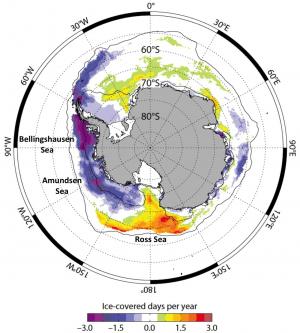
Of course, it’s not that simple. For one thing, the average increase of Antarctic sea ice is actually a small number that is the difference of two big numbers — modest increases over a large area, mostly in the Eastern Hemisphere, and very large decreases over a smaller area in the Western Hemisphere. The map below, showing change in the length of the sea ice season over the last 30 years, illustrates this point well. In spite of the average increase, there are very rapid declines in the Bellingshausen and Amundsen Seas, comparable to sea ice declines in the Arctic. Furthermore, the only season is which there is a significant trend in the westerlies is austral summer. There is a weak positive trend in fall, but both spring and winter show no trend; the SAM trends in these seasons may even be slightly negative, depending on which data are used (Ding et al., 2012). Yet the pattern of sea ice change is quite similar in all seasons: decreasing along the Pacific coast of West Antarctica, and increasing around most of East Antarctica, and in the Ross and Weddell Seas.

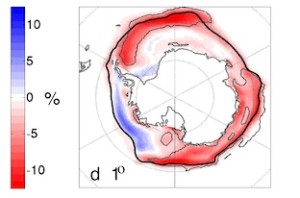
On top of these subtleties, confusion about the role of the winds has arisen because some of the prominent modeling studies that have examined the relationship between the westerly winds and Antarctic sea ice have come up with results that appear to be in direct opposition to the observations. When fully coupled climate models are run with increased CO₂ and decreased stratospheric ozone, the westerly winds increase as has been observed, but sea ice decreases around most of Antarctica. For example, Bitz and Polvani, 2012 found that the pattern of trends is the mirror image of the observations, with increases, rather than decreases in the Amundsen and Bellingshausen Seas.

So what’s really going on? One idea is that changes in ocean stratification might be important. There has been a huge increase in the amount of fresh water getting into the Southern Ocean from melting glaciers, especially in the Amundsen Sea (see, e.g., the latest data from Sutterly et al., 2014). Fresh water forms a sort of buoyant lid on the ocean, limiting the ability of heat from the warmer water below to get to the sea ice and melt it. A study by Bintanja et al. (2013) showed that it was a least plausible that this explains the Antarctic sea ice change. A basic problem, though, is that the greatest discharge of meltwater is occurring in the Amundsen Sea, exactly where sea ice is declining, so while this probably is part of the story, I doubt it’s very dominant.

As it turns out, comparing observations with the results of model experiments like those of Bitz and Polvani (2012) is misleading. Most such experiments are equilibrium experiments: What’s done is to run a model under “preindustrial” conditions, and then to run it again with reduced ozone and increased CO₂, and to look at the difference. This provide a measure of what will eventually happen (at least in the model) after many decades or centuries. But when you look at the transient response to changes in the circumpolar winds, as Marshall et al (2014) have done, it turns out that two important things happen. The winds tend to push the sea ice boundary northward, as we would have expected. But the winds push the surface ocean northward too, and cause a slow rise in the isopycnal surfaces (surfaces of constant density). This brings relatively warm deep water closer to the surface, eventually melting sea ice after a period of a few decades, countering the initial increase in sea ice. These results explain why equilibrium model calculations find sea ice decreasing in response to ozone forced changes in the circumpolar winds, and also why observations show the opposite. Not enough time has passed for the equilibrium response to be manifested. These results suggest that some time in the next few decades, there will reverse, and average sea ice will begin to decline.

Furthermore, there’s a whole lot more going on with the winds than just “increased westerlies”. In the areas where the big sea ice losses have occurred, the concept of “circumpolar westerlies” isn’t very relevant. A far more important measure of wind variability in the Amundsen and Bellingshausen Seas is the Amundsen Sea Low (ASL).⁵ The ASL describes the average location of storms systems the bring heat and moisture into West Antarctica. Changes in the ASL may occur for myriad reasons, but one big hammer that can make it ring is the propagation of atmospheric planetary wave arising out of the tropics, more-or-or less associated with ENSO (El Niño-Southern Oscillation) variability. It’s been clear for many years that ENSO variability play a significant role in sea ice variability in those regions, and recent work shows that this can explain the trends pretty well too (e.g. Yuan and Li, 2008; Stammerjohn et al., 2008). Not incidentally, the adjacent land areas of the Antarctic Peninsula and the West Antarctic Ice Sheet have warmed significantly over the last few decades (Steig et al, 2009; Orsi et al., 2013; Bromwich et al, 2013), and those changes can also be attributed largely to tropical climate variability (Schneider and Steig, 2008; Ding et al., 2011; Schneider et al., 2012; Steig et al., 2013). The cause of temperature and sea ice change is the same: more warm air is being steered into West Antarctica, and the atmospheric flow tends to push sea ice against the continent, keeping it from expanding.

So, do we get the right answer if we take into account all of the wind changes that have occurred over the last few decades? The answer is yes. This is nicely illustrated in a study by Holland and Kwok (2012), who showed that wind, ice motion, and ice concentration changes match each other remarkably well. Where the wind has been increasingly northward, concentrations are increasing; where wind and ice motion changes are toward the continent, ice concentrations are decreasing. And this year, Holland et al. (2014), showed that when they drive an ocean and sea ice model with observed winds — not just increased westerlies, but the full range of wind changes, as calculated by the ECMWF (European Center for Medium Range Weather Forecasting) –- they correctly simulate the overall expansion of sea ice, and they also get the pattern of changes pretty much spot-on. To be sure, the authors note that not all the details are explained, and they highlight the possibly greater importance of thermodynamic consideration (i.e. ocean temperature/stratification) in some areas than in others.  Also, the period they study (1992-2010 only) is pretty short.  The results are nevertheless pretty compelling. Just like the observations, the calculations show large decreases in the Amundsen and Bellinghausen seas, but increases nearly everywhere else.⁷

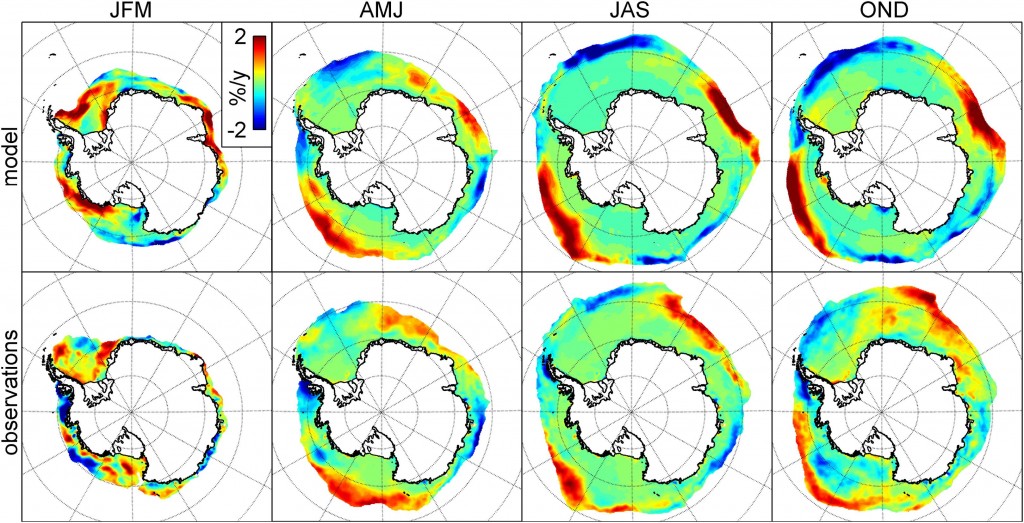
Taken as a whole, these results show that there is no significant contradiction between our understanding of Antarctic sea ice and the observation that it is, in average, expanding. We can explain sea ice trends in the Antarctic rather well if we take into account the full range of changes in winds that have occurred. The average expansion of Antarctic sea ice was not anticipated, but it hardly represents any sort of existential threat to our fundamental understanding of the climate system as a whole. It’s merely an interesting scientific challenge.

Not incidentally, changing winds also have a lot to do with what’s been happening to the Antarctic ice sheet (meaning the land-based glaciers, distinct from the sea ice). I’ll have another post on that later this month, or in the New Year.

For a more in-depth version of this post, see Climate Change National Forum / Making Sense of Antarctic Sea Ice Changes.