Are the heat waves really getting more extreme? This question popped up after the summer of 2003 in Europe, and yet again after this hot Russian summer. The European Centre for Medium-range Weather Forecasts (ECMWF), which normally doesn’t make much noise about climate issues, has since made a statement about July global mean temperature being record warm:
Consistent with widespread media reports of extreme heat and adverse impacts in various places, the latest results from ERA-Interim indicate that the average temperature over land areas of the extratropical northern hemisphere reached a new high in July 2010. May and June 2010 were also unusually warm.
Here, the ERA-Interim, also referred to as ‘ERAINT’, is the ECMWF’s state-of-the-art reanalysis. But the ERAINT describes the atmospheric state only since 1989, and in isolation, it is not the ideal data set for making inferences about long-term climate change because it doesn’t go all that far back in time. However, the statement also draws on the longer reanalysis known as the ERA40 re-analysis, spanning the time interval 1957-2002. Thus, taken into context of ERA40, the ECMWF has some legitimacy behind their statement.
The ERAINT reanalysis is a product of all suitable measurements fed into a model of the atmosphere, describing all the known relevant physical laws and processes. Basically, reanalyses represent the most complete and accurate picture that we can give for the day-to-day atmosphere, incorporating all useful information we have (satellites, ground observations, ships, buoys, aircrafts, radiosondes, rawinsondes). They can also be used to reconstruct things at finer spatial and temporal scales than is possible using met station data, based on physical rules provided by weather models.
The reanalyses are closely tied to the measurements at most locations where observations – such as 2-meter temperature, T(2m), or surface pressure – are provided and used in the data assimilation. Data assimilation is a way of making the model follow the observations as closely as possible at the locations where they are provided, hence constraining the atmospheric model. The constraining of the atmospheric model affect the predictions where there are no observations because most of the weather elements – except for precipitation – do not change abruptly over short distance (mathematically, we say that they are described by ‘spatially smooth and slowly changing functions’).
There are also locations – notably the in the Polar regions and over Africa – where ground-based measurements are sparse, and where much is left for the weather models to predict without observational constraints. In such regions, the description may be biased by model shortcomings, and different reanalysis may provide a different regional picture of the surface conditions. Surface variables such as T(2m) are strongly affected by their environment, which may be represented differently in different weather models (e.g. different spatial resolution implies different altitudes) and therefore is a reason for differences between reanalyses.
Furthermore, soil moisture may affect T(2m), linking temperature to precipitation. The energy flow (heat fluxes) between the ground/lakes/sea and the atmosphere may also affect surface temperatures. However, both precipitation and heat fluxes are computed by the reanalysis atmosphere model without direct constraints, and are therefore only loosely tied to the observations fed into the models. Furthermore, both heat fluxes and precipitation can vary substantially over short distances, and are often not smooth spatial functions.
While the evidence suggesting more extremely high temperatures are mounting over time, the number of resources offering data is also growing. Some of these involve satellite borne remote sensing instruments, but many data sets do not incorporate such data.

All data need to be screened though a quality control, to eliminate misreadings, instrument failure, or other types of errors. A typical screening criterion is to check whether e.g. the temperature estimated by satellite remote sensing is unrealistically high, but sometimes such screening may also throw out valid data, such as was the case of the Antarctic ozone hole. Such post-processing is done differently in analyses, satellite measurements, and reanalyses.
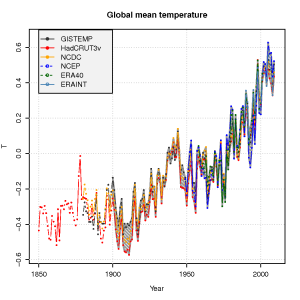
The global mean temperature estimated from the ERAINT, however, is not very different from other analyses or reanalyses (see figure below) for the time they overlap. We also see a good agreement between the ERA40 reanalysis, the NCEP/NCAR reanalysis, and the traditional datasets – analyses – of gridded temperature (GISTEMP, HadCRUT3v, NCDC).
Do the ERAINT and ERA40 provide a sufficient basis for making meaningful
inferences about extreme temperatures and unprecedented heat waves? An important point with reanalyses, is that the model used doesn’t change over the time spanned by the analysis, but reanalyses are generally used with caution for climate change studies because the number and type of observations being fed into the computer model changes over time. Changes in the number of observations and instruments is also an issue affecting the more traditional analyses.
Since the ERAINT only goes as far back as 1989, it involves many modern satellite-borne remote sensing measurements, and it is believed that there are less problems with observational network discontinuity after this date than in the earlier days. It may be more problematic studying trends in the ERA40 data, due to huge improvements in the observational platforms between 1958 and now. Hence, it is important also to look at individual long-term series of high quality. These series have to be ‘homogeneous’, meaning that they need to reflect the local climate variable consistently through its span, not being affected by changes in the local environment, instrumentation, and measurement practices.
An analysis I published in 2004, looking at how often record-high monthly temperatures recur, indicated that record-breaking monthly mean temperature have been more frequent that they would have been if the climate were not getting hotter. This analysis supports the ECMWF statement, and was based on a few high-quality temperature series scattered across our planet, chosen to be sufficiently far from each other to minimize mutual dependencies that can bias the analysis.
The ECMWF provides data for some climate indices, such as the global mean temperature, and the National Oceanic and Atmospheric Administration (NOAA) has a web site for extreme temperatures and precipitation around the world with an interactive map, showing the warmest and coldest sites on the continents. Another useful tool is the KNMI ClimateExplorer, where people can both access data and carry out different analyses on line. It is also possible to get climate data on your iPhone/iPod Touch through Apps like Climate Mobile.
Update: I just learned that NOAA recently has launched a Climate Services Portal on www.climate.gov.
Update: http://rimfrost.no/ is another site that provides station-based climate data. The site shows linear trends estimated for the last 50 years.
Mike Flynn@497
Thanks for your very courteous reply to my ramblings @495. I believe I am struggling with a concept like maybe the laws of Thermodynamics only apply to transfer by conduction where there is thermal equilibrium or for conductive part of convective transfer.
For the bulk motion part of convection transfer(advection) the material itself moves, more governed by Newton’s law, than by thermodynamics. You might get around this explanation by claiming, well there is no equilibrium when matter is being moved. IMO, this is unwise because it sets up an attempt to apply thermodynamics to radiative transfer where equilibrium or non-equilibrium is more difficult to imagine.
Radiative transfer is governed by the laws of electrodynamics, not thermodynamics. Whether you choose to describe the behavior by Maxwell equations (wave theory) or photons (quantum theory) is up to you. In either case I don’t see how thermodynamics is of much use for the transfer.
All that said, I still cop-out; I realize I am, at best, a tyro in these areas.
However, I notice no one has yet tried to answer my question @496 as to how a micro-wave oven works.
I can see how cooking with gas or electricity could be explained by conduction. If one cooks very slowly, thermal equilibrium is pretty close and thermodynamics probably would apply. The heating elements are slightly warmer than the dinner, so energy, in fact, moves from the warmer to the cooler.
But how about a microwave oven? The heating element radiates to the meal, whatever, which is most certainly soon warmer than the “heater”. How does Mike explain that?
It’s not that I disagree with the climate scientist’s explanations, they are most certainly correct. I was hoping to address Mike’s “cold to hot” problem in what I hoped would lead to a simpler explanation, blame it on the transfer type – radiation.
john
Mike Flynn, you sure are having trouble with this. I’ll confine my comment to your response (@484) to my previous post on the topic. I had said that “the atmosphere does not cool–rather, it moderates cooling.”
You replied:
“If the atmosphere doesn’t cool, as you tell me, then the desert surface couldn’t drop below zero at night, after reaching “bloody hot” temperatures during the day.”
Untrue. Take away the atmosphere, and it would cool yet faster than it does now, since there would be zero back radiation to offset the radiated heat.
In fact, that is precisely what happens on the Lunar surface. (A contrasting case would be a situation with a clear sky, but very high humidity; cooling will be much slower, since water vapor is a potent GHG.)
Think hard about that, and most of your troubles understanding this topic will go away. (Not all: you remark to Ray that: “What people seem to forget is that any energy absorbed by the atmosphere, is energy that didn’t reach the surface directly.” But I’ll leave you to ponder why that is also incorrect.)
MF 484,
You are restating my experiment and changing the inputs. The granite blocks effectively only have ONE side, not SIX. The backs have zero emissivity. The sides are too thin to matter. I said so, did I not? Read it again.
A colder body can indeed warm up a warmer body. The second law of thermodynamics says that NET heat cannot be transferred from cold A to warm B. It does NOT say that NO heat can. Have warm B with nothing around it, then introduce cold A which is giving off infrared near B, and B will warm up. Period.
To think otherwise is to think that an inanimate object can somehow tell whether the IR photon it is receiving comes from a warmer or a cooler object. It can’t.
MF 489: Your statement that surface would be cooler if the CO2 was not there, doesn’t seem to agree with the observation that the surface of the moon, without the assitance of CO2, gets hotter than that of the Earth.
BPL: Not on average, it doesn’t.
Flux density absorbed by a planet is
F = (S / 4) (1 – A)
where S is the solar constant and A the bolometric Russell-Bond spherical albedo. For the Moon, we have S = 1366.1 watts per square meter, and Bonnie Buratti’s group says A = 0.11. That gives F = 304 Watts per square meter. Inverting the Stefan-Boltzmann law:
Te = (F / σ)^0.25
where σ is the Stefan-Boltzmann constant (5.6704 x 10^-8 W/m^2/M^4 in the SI). This gives the Moon a radiative equilibrium temperature of 271 K.
For the Earth, A = 0.306, F = 237 W/m^2, and Te = 254 K. Colder! But at the SURFACE, the Earth averages 288 K.
Where do you think the extra 34 K comes from?
MF 497: I don’t believe I’m confused about the laws of thermodynamics to the point where a cooler body can heat a warmer body (in any meaningful sense above the quantum level).
BPL: That is exactly what you’re confused about. A colder body CAN heat a warmer body. You still confused net heat with heat.
When photons strike absorbing material, does the absorbing material heat up? Yes or no?
QM is useless to this discussion. Classical physics, thermodynamics explains heat transfer. Heat transfers from one body to another both warming and cooling bodies.
@498 Anonymous Coward
I notice you haven’t deigned to my answer my question. I understand. I see your response was to advise John Peter about my “error”. Maybe John Peter will read what I said, and ask me to respond. He, of course, may choose not to.
[Response: OK, fool me once shame on you, fool me twice, shame on me. I see that you’re interested in word games and arguing with everyone in sight, and we don’t have time or patience for that, sorry.–Jim]
[edit]
Re 502 John Peter –
The laws of thermodynamics do apply to radiation;
at a given frequency and direction (and polarization, if necessary), the intensity of radiation can be ascribed a brightness temperature, that corresponding to a blackbody which would emit such an intensity of radiation and thus be in equilibrium with that radiation.
Even when the (non-photon) matter is not in equilibrium with the radiation (photons), so long as the matter, or some subset of it, is at LTE, then in a sufficiently small volume (to be approximately isothermal over space) that subset of matter has an energy distribution characteristic of a temperature
and,
for a given frequency, direction, and polarization, it will absorb (over a given path length) some fraction (absorptivity) of incident radiation intensity and emit some intensity (from along the path length that reaches the end of the path length) into that direction that is the same fraction (emissivity) of the blackbody value for the temperature of the matter; thus,
emissivity = absorptivity, and if the temperature of the matter is greater than, equal to, or less than the brightness temperature of the incident radiation, it emits more, the same, or less of that type of radiation into that direction than it absorbs from that direction.
Also
for (non-photon) matter at LTE (and setting aside relativistic effects, and assuming photon energy is conserved upon any scattering/reflection),
considering the contributions of emission from different places (see ’emission weighting function’) that add up to the incident radiation arriving at any one location, one can find that the fraction of radiation emitted from one place that reaches and is absorbed in another is the same as the fraction of radiation that is emitted in the second place which reaches and is absorbed in the first (for conditions that are not changing over the time it takes for the radiation to get from one place to another, which is a safe approximation for exchanges within the climate system); thus, the net radiant heat flux (the difference between fluxes in opposite directions), from emission to absorption, exchanged between two volumes of matter, is from a warmer volume to a colder volume; and the emission and absorption of that radiant heat contribute to the radiant cooling and heating of non-photon matter.
…
Of course, the laws of thermodynamics do not by themselves simply dictate what fraction of radiation from point A reaches point B, or what the emissivity and absorptivity are (except that they are equal to each other for particular circumstances). That is where optical properties and the physics of electromagnetic radiation come in to it.
…
PS refraction can concentrate or expand radiation to a narrower or wider range of directions, thus changing the intensity. However, blackbody radiant intensity changes the same way, so brightness temperature is conserved absent emission, absorption, or scattering/reflection, and the net flow of radiation is still from warmer to cooler material (for the conditions mentioned above).
…
The flow of heat carries energy and entropy, where the flux of entropy = the flux of energy (in the form of heat) divided by the temperature. Thus when the same net flux of heat leaves a warm body and enters a cooler body, the entropy gain by the cooler body is larger than the loss from the warmer body; there is a net gain of entropy associated with such spontaneous flow of heat (not surprisingly).
Of course,
heat that carried with a flow of matter tends to be toward a lower concentration of matter; aside from convection across a temperature gradient within the material, such a flux of heat may occur due to effusion or diffusion (as with latent heat of some substance), in which case it is possible for heat to flow toward higher temperatures, because the entropy gain from the mixing of material may offset the loss from the flow of heat to higher temperature.
Aside from that,
conserving entropy would require a flow of heat from/to a warmer material to be larger than that entering/leaving a cooler material, and the difference is what is available to be converted to work in a heat engine, or what is the minimum amount of work required to run a corresponding heat pump.
Regarding radiation,
radiation carries entropy according to it’s brightness temperature (energy/temperature); radiation from the sun has less entropy (per unit energy) than radiation emitted from the Earth. There is entropy gain when solar radiation is absorbed by the cooler Earth, when radiation is exchanged among layers with different temperatures,
and also
when convection occurs (with some heat (in the form of available potential energy (internal energy + gravitational potential energy (which is associated with heat because of thermal expansion in a hydrostatic fluid)) converted to work when warm air rises and cooler air sinks (thermally-direct convection), and some of that work converted back to heat by the reverse type of motion (thermally-indirect convection); heat can also drive motion by altering composition (phase changes of water and associated concentration of impurities in the ocean (important), changes in air density (minor effect), to supply available (gravitational) potential energy that can be converted to kinetic energy, which can be converted back to graviational potential energy; and then (covering almost all the bases, though this is quite minor relative to the total fluxes) there’s conversion to chemical energy (ozone, photosynthesis, some geochemical stuff (involves geothermal energy too, which also is converted to graviational potential energy via raising mountains, etc, which then is converted to kinetic energy during erosion, which then…) – this is quite minor relative to the kinetic energy production, which is a small fraction of the total energy flux through the climate system –
…
anyway, all that kinetic energy which doesn’t get converted back to heat energy in another way is converted to heat by viscosity (friction).
…
To sum up, in equilibrium, the energy fluxes in and out are balanced; the entropy fluxes in and out are different but are balanced by entropy production.
Regarding microwaves: I don’t know all the details but there is a device which converts electrical energy (work) to electromagnetic radiation. I would presume, in analogy to radio antenna, that the radiation has very low entropy. Work can in principle be converted to heat (directly 1-to-1 conversion, as opposed to a heat pump) at any temperature or radiation at any brightness temperature; any temperature less than infinity involves some entropy, so such a conversion always involves some gain in entropy.
PS Laser radiation has very low entropy because a flux of energy is concentrated into a narrow range of directions (high intensity) and frequencies (high spectral intensity, therefore high brightness temperature) and phases and (I would guess) polarizations (coherent vs incoherent radiation, polarized vs unpolarized; how hot would blackbody radiation have to be to have the same intensity within any such narrow range of phases and polarizations – I presume that is the true brightness temperature of such radiation).
Radiation from a single antenna is not concentrated in direction but is (or can be) concentrated in the other ways (polarized, monochromatic, coherent). Although in a microwave, I think the waves are scattered a bit before entering the food, so it may not be polarized and coherent at that point, but still monochromatic?). It only takes concentration to a single value in any one dimension to produce zero entropy, as I understand it (am I wrong?).
(PS if my understanding is correct, a range of frequencies of radiation may be absorbed by a fluorescent material with some of that energy emitted nearly monochromatically; such nearly monochromatic radiation (if of significant intensity) could have a very high brightness temperature, and thus some entropy must be left behind in the material in order not to violate the second law of thermodynamics (it is applicable even in non-LTE conditions). Flourescence as a heat engine? or maybe heat pump?)
So most of the energy in such radiation is available to do work. But it can also simply be absorbed as heat. Microwave radiation is at a frequency that can easily excite water molecules in particular, giving them kinetic energy.
“Radiation from a single antenna is not concentrated in direction”
… well, it’s not isotropic, but it’s no laser beam either.
“one can find that the fraction of radiation emitted from one place that reaches and is absorbed in another is the same as the fraction of radiation that is emitted in the second place which reaches and is absorbed in the first”
As stated this isn’t correct; it just needs a little adjustment. (to be cont.)
Mike Flynn (489): “…the cooler body cannot increase the net energy of the warmer…” tis true. But it can add gross energy to the warmer body.
A perfect reflector, (not an insulator), would radiate back to the emitter 100% of the emitted radiation of all wavelengths. True again, this would not make the emitter hotter than it was originally. But it will make the emitter warmer that if the reflector wasn’t there.
To 273 Edward Greisch: As a retired Federal employee who happily called herself a “bureaucrat” throughout her career, I want to say, “Thank you.”
Polarization has nothing to do with infrared. Polarizers are very sensitive scientific instruments. We do not have KCL windows for the planet. Blackbody temperature is not helpful in this particular topic. In pyrotechnics brightness temperature is used like in comparing sparks; the darker the sparks the lower the temperature.
The physics of temperature is not so convoluted as that.
Viscosity has nothing to do with it. Heat goes to a cooler body.
BPL your physics discussion has nothing to do with this discussion or any of the off topic ones precisely either so it is very off topic.
BPL: That is exactly what you’re confused about. A colder body CAN heat a warmer body. You still confused net heat with heat.
No this is false. You are making a statement that violates the first law and parts of the second too.
We need energy to compress a gas or heat energy to drive a cooling process. In an air conditioner or refrigerator you need electrical energy to perform the work. You cannot move in the opposite direction of thermodynamics. You can have adiabatic expansion moving the molecules farther apart to cool and then it draws the heat out of the refrigerated space. Compression does not occur by itself. In statistical thermodynamics even if go below aboslute zero we are not at equilbrium so we do not violate thermodynamics. Caloric theory is false so heat cannot go from a cooler body to a hotter body.
Actually in this case your statement violates all 4 laws of thermodynamics.
Recommended reading, from before the politicalization of climate change:
Steiger, Brad, “A roadmap of time: How the Maxwell/Wheeler weather-energy cycles predict the ‘history’ of the next 25 years” Prentice-Hall, 1975
If Steiger’s analysis is right, we are at the peak of a “warm-dry” phase (and that produced the measurements under discussion), but we should be shifting into a cold-dry phase this decade. Please consider.
[Response: This Brad Steiger? – really? – gavin]
Jacob 514:
None of this discussion makes any sense without reference to heat flux. Reducing the flux of heat out of a heat producing body will generally result in a temperature increase unless the body can control the rate at which it produces heat. In short; insulators decrease heat flux and raise temperatures. Essentially all of the discussion above applies equally well to thermal conduction as to radiation. Most people put on more blankets when they’re cold. If the blankets are initially cold they will still warm the person up but first the blankets will be warmed up. The blankets themselves will remain cooler that the person’s skin temperature. Is this an example of a cooler object warming a warmer object? Not really. Only a person who was being deliberately obfuscatory would make such claims.
Here is a simple model for conduction of heat away from a human covered by blankets.
T_body = person’s internal body temperature
s = the thickness of the person’s skin
ks = thermal conductivity of human skin
T_skin = temperature at the surface of the person’s skin
B = thickness of the blankets the person is using
kb = thermal conductivity of the blankets
T_room = temperature of the room the person is sleeping in.
Heat_flux_skin = heat flux through the skin
Heat_flux_blankets = heat flux through the blankets
Heat_Flux_skin = (ks/s) (T_body-T_skin)
Heat_Flux_Blankets = (kb/B) (T_skin-T_room)
The body controls the body temperature through metabolic processes and it fair to assume it is constant under healthy conditions. The room temperature is similarly controlled by thermostats and the temperature outside, etc. What isn’t controlled is the skin’s surface temperature: T_skin. Normally T_room < T_skin < T_body .
At steady state the heat flux through the skin equals the heat flux through the blankets. When you pile on blankets the skin temperature will increase until these fluxes are equal. It doesn't matter if the blankets were initially cold. The steady-state skin temperature is given by:
T_skin = T_body / (1 + R) + T_room R /(1+R)
where R = kb s /(ks B)
As more and more blankets are piled on B gets larger and larger so that R becomes smaller and smaller and the warmer the person gets. The best they can do is to lose zero heat to conduction by using a very thick pile of blankets.
The temperature profile in the blankets is given by:
T_blanket(z) = T_skin – (T_skin-T_room) z/B
where z is the distance from the skin (z=0 is at the skin, z=B is at the room). The blanket is lower than the skin temperature everywhere. Yet increasing the the blanket thickness results in increasing the body temperature. I don't know how to make this any clearer (although I'm sure someone can).
Re 514 Jacob Mack Polarization has nothing to do with infrared.
I was just covering my bases.
So far as I know (?) you can pretty much ignore polarization for LW (terrestrial) radiation (wavelengths longer than about 4 microns, in constrast to SW radiation) for Earth’s surface and atmosphere without much error, because LW scattering is minor and emission and absorption by randomly-oriented gas molecules and spherically-symmetric cloud droplets will be unpolarized. However, cirrus ice crystals sometimes float in preferred orientations, so…? Of course, if a process emits polarized radiation or scatters radiation in different directions depending on polarization (thus able to produce polarized radiation from unpolarized radiation) or absorbs or reflects or transmits depending on polarization (thus able to produce polarized radiation from unpolarized radiation), but then the subsequent interactions (to the point of absorption or escaping the system) is independent of polarization, then the polarization can be ignored.
Blackbody temperature is not helpful in this particular topic.
Are you talking about the original topic of extreme weather. Okay (although in the context of climate change, it could still come up). But it certainly is helpful – is key – in discussing thermodynamics of radiation.
In pyrotechnics brightness temperature is used like in comparing sparks; the darker the sparks the lower the temperature.
The physics of temperature is not so convoluted as that.
Well, if you have a process that is not sensitive to any other characteristics besides whole-spectrum radiant intensity, than you could assign a brightness temperature for that intensity for that context. Likewise if a process is not sensitive to polarization, one can take the intensity summed over all polarizations, etc.
Viscosity has nothing to do with it. Heat goes to a cooler body.
That came up because I went on to discuss the entropy production of the climate system. Some heat is converted to kinetic energy via heat engines (thermally-direct motions); some is converted back to heat via heat pumps (thermally-indirect motions), but some is converted back to heat by friction.
Actually in this case your statement violates all 4 laws of thermodynamics.
BPL was not asserting that *the* (ie net) flux of heat was up-gradient, or that energy was being created, or that conversion efficiencies of either heat engines or heat pumps (or any radiant, electrochemical, etc, process with the same effect) could exceed those limits determined by the inability to destroy entropy. He was stating that a cold body can keep a warm body warmer than it otherwise would be by hiding the warm body from an even colder body or something equivalent (the darkness of space) – ie
a winter coat or blanket will generally be colder than human body temperature (even just at the skin) but still slows the rate of heat loss (impeding radiation, conduction, and convection (sensible and latent)) from a human body to a colder environment, allowing the heat output from metabolism to build up more near the skin and thus raising skin temperature.
The greenhouse effect of the atmosphere works the same way on LW radiation, which is, in the global time average, the way nearly all heat leaves the surface+troposphere (as a single unit) and the only significant way heat escapes to space.
Jacob Mack, I fear I have to agree with BPL, because the “doing work” thing is a side issue in his example. (And his example is quite instructive, IMO.)
If you have a body A at 400 degreesK and it is radiating away the S-B calculated energy, and say there is a body B that, by our experiment, is radiating a narrow beam of energy at A exactly equal to A’s emission, then A remains at its thermo equilibrium 400 degrees.
Now move body C at 200 degreesK into the two-body system. (And the work involved with moving body C or heating it up earlier is not relevant.) It is also radiating energy per S-B. Some of 200-degree C’s emitted radiation will be absorbed by 400-degree B, and B’s temperature will rise. B will emit no more radiation than it was in the first instance, i.e. its radiation will not change one iota at time zero when C enters the picture — its radiation is determined entirely by its own temperature. Then after B’s temp begins to rise because of cooler C’s radiation, it will increase its radiation because it is now getting warmer from cooler C’s emission.
Thanks to everyone who has tried help me understand some Radiation Thermodynamics. Consider your posts as yet another example of the wide ranging value of RealClimate as a teaching tool.
Being pretty well snowed, I would like to pursue my own education through some textbook. I note there is a relatively recent text – “Engineering Thermodynamics of Thermal Radiation” by Richard Petela
Would this be a good starting point for this tyro who doesn’t yet see how to treat the thermodynamics of photons? Any better suggestions – texts or papers?
TIA
516 Patrick 027: As you can see we are in complete agreement (see pearson 516) regarding the physics. That being said I think Barton used a poor choice of words when he referred to \net energy.\ Rod B used excruciatingly bad terminology in his post (512) in which he stated that a cooler body can \add gross energy\ to a warmer body. These discussions can be made with precision. Precision requires explicit mention of heat fluxes. ( I know you know all this but I thought it worth saying anyway.) The earth can be considered a heat source. It can be considered a heat source because the atmosphere is transparent to short wave length radiation which comes from the sun. Sunlight passes through the atmosphere hits the surface and is re-radiated at much longer wavelengths (and cooler temperatures). This is exactly analogous to a person who is in bed and metabolizing and producing heat and using a blanket to stay warm.
There is an outward flux of long wavelength light called \infrared radiation\ emitted by the earth’s surface just as their is an outward flux of heat from a person. The greenhouse gases (CO2 and water vapor) in the earth’s atmosphere absorb the outward long wave radiation emitted by the earth’s surface. The absorbed radiation is re-emitted by the greenhouse gases uniformly in all directions. Half of that radiation goes down. Half goes up. Estimation of the surface temperature requires consideration of all heat fluxes in and out at the surface. On average, the gases in the troposphere (where we live and where weather happens) are cooler than the earth’s surface. The heat fluxes at the surface include not only the primary radiation coming from the sun but also the radiation that is re-emitted by the earth’s atmosphere. The proper conclusion is that increasing the thickness of the blanket of gases surrounding the planet results in a warmer surface temperature exactly analogous to the way that a blanket functions to keep a person warmer. In both cases the blanket and the blanket of gas are cooler than the surface temperature and this is all in accord with physics that has been understood for nearly 200 years. There are lots of details regarding the best estimates of how much the temperature will increase from a given CO2 increase but the basic physics is beyond dispute.
BPL: That is exactly what you’re confused about. A colder body CAN heat a warmer body. You still confused net heat with heat.
JM: No this is false. You are making a statement that violates the first law and parts of the second too.
BPL: I always like it when an amateur scientist says that I, a guy with a degree in physics, got the elementary physics wrong. Read and learn, JM:
http://bartonpaullevenson.com/JJandJ.html
John E. Pearson, I was trying to explain a limited simple concept to Mike Flynn about heat transfer from a cooler body to a warmer body. I made no attempt to explain molecular absorption or all of climatology. In that context “added gross energy” is not a faulty phrase and describes the limited process aptly.
I have some criticism of your words in #520, though they’re probably not helpful in the context of your overall thought. However, I am curious: what is meant by “exactly analogous?” Also, I think the inside of the blanket equalizes at the body temperature.
522 Rod B: Please don’t start with another one of your tarbabies. I don’t know if it was deliberate but your discussion regarding gross and net energy was obfuscatory.
The temperature near the outside of your skin in contact with air is lower than body temperature. Get a thermometer and try it yourself. If you put the thermometer under your arm and mash your arm down on the thermometer you can get it up to body temperature. If you stick the thermometer on your leg under a blanket it will show a lower temperature. Try it. If you’re using so many blankets that the temperature inside the blanket is body temperature you’re going to be VERY uncomfortable.
John E. Pearson, “gross” and “net” are pretty rudimentary and simple concepts to most. Nothing obfuscating about them though might not be pedantic enough for you. I meant “gross” as the total energy entering a body; “net” as the total energy entering a body less the energy leaving the body. I am simply supporting BPL with what might be terms easier for MF to grasp. I didn’t start it; go fuss at MF or BPL for generating tarbabies.
Is the temperature of the inside of the blanket warmer, cooler, or the same as the “surface” temperature of the body? You initially said cooler. Sticking with that?
Rod please do not try to obfuscate. I did remark that Barton used poor terminology. Your terminology was worse than Barton’s.
I don’t know what you mean by the “temperature inside of the blanket”. Here I will once again try to make the discussion precise. At steady state the temperature at any point in a blanket will be cooler than the body temperature of the person beneath the blanket and warmer than room temperature. If that is what you’re asking me if I am sticking with the answer is; “Yes. I am sticking with it.” Get a thermometer and try it. I did. I put the thermometer in my mouth. It read 98.6F. I put it under my arm and it read 95F. I pressed the thermometer against my bare leg which was covered by my blanket. It read 90F. I put the thermometer on top of the blanket and the temperature (probably 65-70F) was below the bottom scale on my oral thermometer so it didn’t register. I took the blanket off and felt chilly. I pressed the thermometer agains my bare leg and it didn’t register because the temperature was below scale. I put the blanket back on and waited until I warmed up again. I pressed the thermometer against my bare leg. it read 91F. Learn a little physics (transport theory). Make some measurements. Think. This really needn’t be complicated.
I wrote:
“The temperature profile in the blankets is given by:
T_blanket(z) = T_skin – (T_skin-T_room) z/B
where z is the distance from the skin (z=0 is at the skin, z=B is at the room). The blanket is lower than the skin temperature everywhere. Yet increasing the the blanket thickness results in increasing the body temperature.”
the final “body” (second to last word) was a typo and should’ve been “skin” meaning the temperature measured at the skin. The temperature measured at a person’s on the surface of a person’s skin is not generally body temperature. It is cooler. Don’t take my word for it. Measure it. If you don’t like my model of how a blanket functions to keep a person warm that is fine. There are some quibbles that could be raised but the physics is essentially correct and stated with reasonable precision. If you insist there is a major flaw then state what the flaw is. Be precise. Do try not to obfuscate.
Re Rod B , John E. Pearson – you might not be aware of the history of the term ‘tar….’ – I’d advise not using it; maybe use the phrase ‘glue ball’ ?
I thought Rod B’s 518 was good.
The temperature may vary continuously through the blanket-skin contact (where they are in contact); anyway the blanket on average is colder and removing colder layers of the blanket will result in a decrease in temperature of the rest of the blanket and then of the skin as heat is lost faster.
John Peter at 519
“Spectrophysics” by A.P.Thorne is a nice text but don’t know if it’s still in print.
Michael@527
Thanks. Looks really good, I’d never thought to get at radiation thermodynamics through spectroscopy but it should be a much more satisfactory path for me.
The book is not currently available from Amazon. I put it on my list.
Thanks again,
John
Patrick 027, you’re right about “tar___.” Just forgot. My bad.
PAtrick 027 526: Rod’s 518 was ok. It wasn’t as bad as the post that I was discussing which was 512. Regarding tar babies I’ve used that phrase for 50 years and am well-aware of the history. “Tar baby” is an expression meaning something you get stuck in and the more you struggle to free yourself the more stuck you get. In my view Rod is a master of internet tar babies. In any event I see no reason to stop using that phrase now. My guess is that you’re not as aware of the history of the phrase as I am. http://en.wikipedia.org/wiki/Joel_Chandler_Harris I grew up in the south. Not the deep south but northern Virginia. Mrs Squires, our librarian (at Stonewall Jackson Elementary School) read Harris to us from kindergarden on. She explained the history and how it was that a white man had archived these stories that had been passed down by slaves. I read Harris to my two older children before I read them Mark Twain. I will read him to my youngest after she is born. I would no more dismiss Harris than I would Mark Twain. I know there were movements to have both of them removed from public libraries and school libraries starting at least by the mid 1970’s, maybe even earlier. In my view these movements were misguided.
Re 520 John E. Pearson –
The absorbed radiation is re-emitted by the greenhouse gases uniformly in all directions. Half of that radiation goes down. Half goes up.
Yes, for a sufficiently thin layer; when that radiation can be absorbed again before escaping the atmosphere, the emissions from different parts of the atmosphere don’t all reach the surface or space equally; the downward flux from the atmosphere will tend to be larger than the upward flux from the atmosphere to space because of the general decrease in temperature with height (in particular as measured by optical thickness).
The proper conclusion is that increasing the thickness of the blanket of gases surrounding the planet results in a warmer surface temperature exactly analogous to the way that a blanket functions to keep a person warmer.
In the global average that’s how it works above the tropopause to a good approximation, convective fluxes being almost zero. OLR at TOA is reduced by increased greenhouse forcing, and warming must occur somewhere below TOA to restore OLR to balance solar heating. The same (for OLR) is approximately true for net upward LW flux at the tropopause or anywhere above that (to balance the forcing remaining after stratospheric adjustment plus any feedbacks). Below that, convection can respond to changes in radiation. Generally, convection tends to maintain a particular lapse rate (which itself is climate dependent) which determines where how the warming is distributed below the tropopause. Of course, in some conditions and locations (nocturnal inversions, high latitudes – winter in particular), surface temperature may respond to radiation without such a convective response – though there is still horizontal transport locally that is connected to the larger-scale overturning of the troposphere. And then there’s changes in soil moisture and winds, etc, that can affect the relationship between surface and near-surface air temperature, etc. But that’s more ‘second-order’ stuff, as I understand it. (While the radiative forcing at the tropopause level is key to large scale warming of the surface and tropopshere in general, the effect of radiation at the surface is important to the diurnal temperature range over land.)
There are lots of details regarding the best estimates of how much the temperature will increase from a given CO2 increase but the basic physics is beyond dispute.
I think that’s a fair statement.
Stastical thermodynamics proves why thermodynamics cannot be violated at the micro-level. The correspondence principle.